CBSE Class 10 – Mathematics Basic Previous Paper 2023
MATHEMATICS (BASIC)
Time allowed: 3 hours
Maximum Marks: 80
General Instructions:
Read the following instructions carefully and follow them:
-
This question paper contains 38 questions. All questions are compulsory.
-
Question Paper is divided into 5 Sections – Section A, B, C, D, and E.
-
In Section–A, questions 1 to 18 are Multiple Choice Questions (MCQs), and questions 19 & 20 are Assertion-Reason based questions, each carrying 1 mark.
-
In Section–B, questions 21 to 25 are Short Answer-I (SA-I) type questions, each carrying 2 marks.
-
In Section–C, questions 26 to 31 are Short Answer-II (SA-II) type questions, each carrying 3 marks.
-
In Section–D, questions 32 to 35 are Long Answer (LA) type questions, each carrying 5 marks.
-
In Section–E, questions 36 to 38 are Case-Based integrated units of Assessment questions, each carrying 4 marks. Internal choice is provided in the 2-mark question within each case-study.
-
There is no overall choice. However, internal choices are provided as follows:
- 2 questions in Section B
- 2 questions in Section C
- 2 questions in Section D
- 3 questions in Section E
-
Draw neat figures wherever required. Use π = 22/7 if not specified otherwise.
-
Use of calculator is NOT allowed.
Section – A
(Multiple Choice Questions)
This section consists of 20 questions, each carrying 1 mark.
-
The prime factorization of natural number 288 is:
- (a)
- (b)
- (c)
- (d)
Answer: (c)
- (a)
-
If
, then the value of
is:
- (a) 45°
- (b) 60°
- (c) 30°
- (d) 90°
Answer: (c) 30°
-
A card is drawn at random from a well-shuffled deck of 52 cards. The probability of getting a red card is:
- (a)
- (b)
- (c)
- (d)
Answer: (d)
(There are 26 red cards out of 52 cards.)
- (a)
-
The discriminant of the quadratic equation
is:
- (a) 1
- (b) 49
- (c) 7
- (d) 19
Answer: (b) 49
(Discriminant ( \Delta = b^2 – 4ac = (-5)^2 – 4(2)(-3) = 25 + 24 = 49.)
-
The distance between the points (3, 0) and (0, –3) is:
- (a)
units
- (b) 6 units
- (c) 3 units
- (d)
units
Answer: (b) 6 units
- (a)
(Distance =
=
=
=
units.)
-
The seventh term of an A.P. whose first term is 28 and common difference –4, is:
- (a) 4
- (b) 44
- (c) 52
- (d) 56
Answer: (a) 4
*(The
-th term of an A.P. =
.
For the 7th term:
)
-
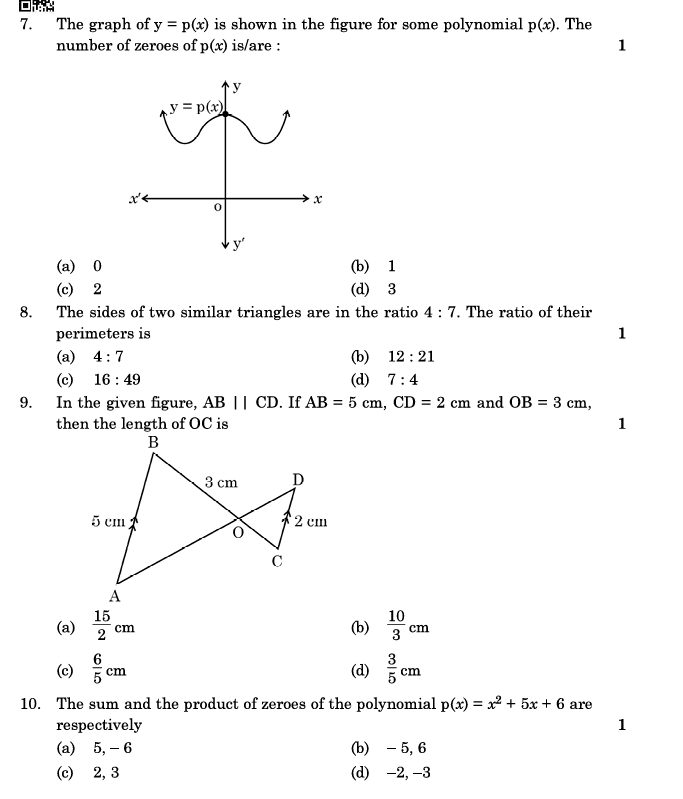
The graph of
is shown in the figure for some polynomial
. The number of zeroes of
is:- (a) 0
- (b) 1
- (c) 2
- (d) 3
Answer: (d) 3
(The graph intersects the x-axis three times, indicating 3 real zeroes.)
-
The sides of two similar triangles are in the ratio 4 : 7. The ratio of their perimeters is:
- (a) 4 : 7
- (b) 12 : 21
- (c) 16 : 49
- (d) 7 : 4
Answer: (a) 4 : 7
(The ratio of perimeters of similar triangles is the same as the ratio of their corresponding sides.)
-
In the given figure,
. If
cm,
cm, and
cm, then the length of
is:- (a)
cm - (b)
cm - (c)
cm - (d)
cm
Answer: (b)
cm - (a)
(Using properties of similar triangles and proportional segments.)
- The sum and the product of zeroes of the polynomial
are respectively:
- (a) 5, –6
- (b) –5, 6
- (c) 2, 3
- (d) –2, –3
Answer: (b) –5, 6
*(For
, sum of roots =
and product of roots =
)
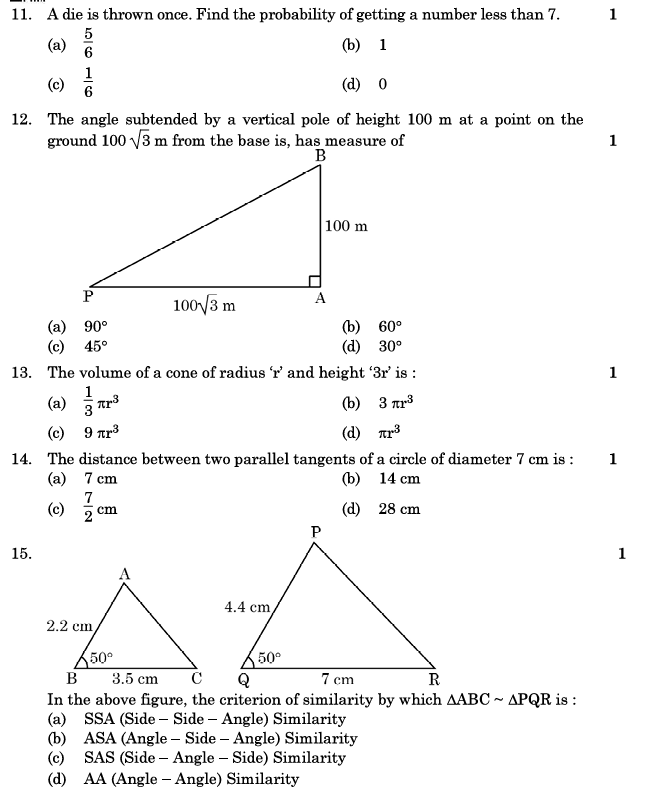
11. A die is thrown once. Find the probability of getting a number less than 7:
- (a)
- (b) 1
- (c)
- (d) 0
Answer: (b) 1
(All 6 possible outcomes (1, 2, 3, 4, 5, 6) are less than 7, so
.)
12. The angle subtended by a vertical pole of height 100 m at a point on the ground
m from the base is:
- (a) 90°
- (b) 60°
- (c) 45°
- (d) 30°
Answer: (b) 60°
(Use trigonometry:
, giving
.)
13. The volume of a cone of radius
and height
is:
- (a)
- (b)
- (c)
- (d)
Answer: (b)
(Formula:
.)
14. The distance between two parallel tangents of a circle of diameter 7 cm is:
- (a) 7 cm
- (b) 14 cm
- (c)
cm - (d) 28 cm
Answer: (a) 7 cm
(Distance between parallel tangents = diameter = 7 cm.)
15. In the figure, the criterion of similarity by which
is:
- (a) SSA (Side–Side–Angle) Similarity
- (b) ASA (Angle–Side–Angle) Similarity
- (c) SAS (Side–Angle–Side) Similarity
- (d) AA (Angle–Angle) Similarity
Answer: (c) SAS (Side–Angle–Side) Similarity
(Two sides are proportional and the included angle is equal.)
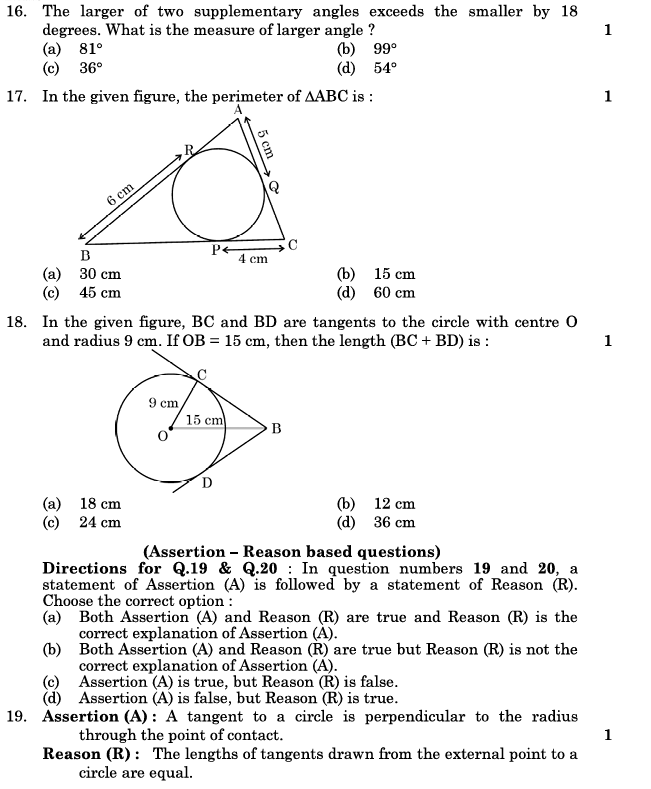
16. The larger of two supplementary angles exceeds the smaller by 18°.
- (a) 81°
- (b) 99°
- (c) 36°
- (d) 54°
Answer: (b) 99°
(Supplementary angles sum to 180°. Let the smaller angle be
. The larger is
. So:
(smaller), larger =
.)
17. In the given figure, the perimeter of
is:
- (a) 30 cm
- (b) 15 cm
- (c) 45 cm
- (d) 60 cm
Answer: (c) 45 cm
(The perimeter is calculated using tangent properties and given lengths.)
18. In the given figure,
and
are tangents to the circle with centre
and radius 9 cm. If
cm, then the length
is:
- (a) 18 cm
- (b) 12 cm
- (c) 24 cm
- (d) 36 cm
Answer: (c) 24 cm
(Tangents from an external point are equal, so each tangent has length
. So,
cm.)
Assertion-Reason Based Questions
19.
Assertion (A): A tangent to a circle is perpendicular to the radius through the point of contact.
Reason (R): The lengths of tangents drawn from the external point to a circle are equal.
- (a) Both A and R are true and R is the correct explanation of A.
- (b) Both A and R are true but R is not the correct explanation of A.
- (c) A is true, R is false.
- (d) A is false, R is true.
Answer: (b) Both A and R are true, but R is not the correct explanation of A.
(The tangent is perpendicular to the radius due to circle properties, but that isn’t explained by the equality of tangent lengths.)
20.
Assertion (A): The sum of the areas of two circles with radii
and
is equal to the area of a circle with radius
.
Reason (R):
.
- (a) Both A and R are true and R is the correct explanation of A.
- (b) Both A and R are true but R is not the correct explanation of A.
- (c) A is true, R is false.
- (d) A is false, R is true.
Answer: (a) Both A and R are true and R is the correct explanation of A.
(Area of a circle:
. So:
, hence
.)
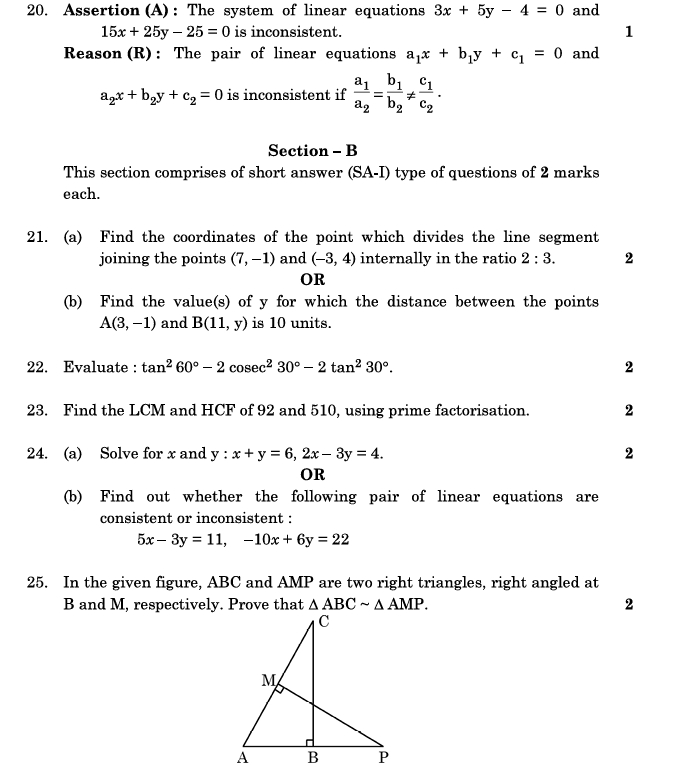
20. Assertion & Reason
Assertion (A): The system of linear equations
and
is inconsistent.
Reason (R): The system
and
is inconsistent if:
- (a) Both true, R explains A.
- (b) Both true, R does not explain A.
- (c) A true, R false.
- (d) A false, R true.
Answer: (a) Both true, and R explains A.
(After simplifying, the two lines are parallel but distinct, which makes the system inconsistent.)
21. Coordinate Geometry
(a) Find the point dividing the segment joining
and (-3, 4) **in the ratio** \( 2:3.
Formula:
Answer:
.
22. Evaluate:
Now calculate:
Answer:
.
23. Find the LCM and HCF of 92 and 510.
Prime Factorization:
HCF:
(only common factor)
LCM:
.
Answer: HCF = 2, LCM = 23460.
24. Solve:
From
, substitute into
:
Answer:
.
25. Prove
.
- Both are right-angled triangles (angle =
)
(corresponding angles equal)
(proportional sides)
Answer: By AA Similarity Criterion.
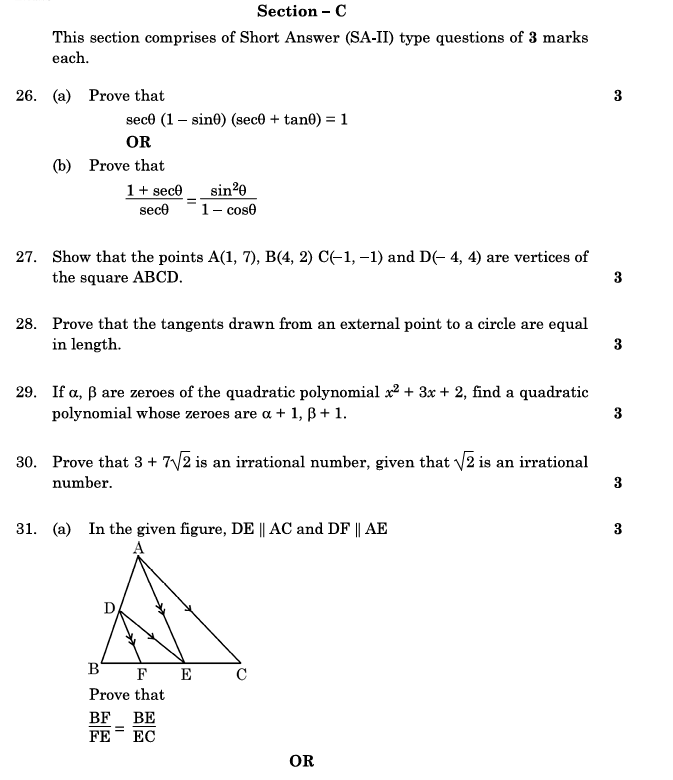
26. (a) Prove:
LHS:
Hence, proved. ✅
27. Show points
are vertices of a square.
Use distance formula
:
- AB:
- BC:
- CD: Similarly,
- DA:
All sides are equal, and diagonals are equal.
Hence, it’s a square. ✅
28. Prove: Tangents from an external point are equal.
Let
be the external point and
&
the points of tangency. Join
(center) with
,
, and
.
(radius)
is common.
by RHS criterion.
Thus,
.
Proved. ✅
29. If
are roots of
, find a polynomial with roots
.
Given:
New roots:
.
Sum of new roots:
Product:
Polynomial:
Answer:
. ✅
30. Prove
is irrational (given
is irrational).
Assume
is rational. Let
(rational).
The right side is rational, but the left side
is irrational. Contradiction.
Hence,
is irrational. ✅
31. Prove:
when
and
.
By Basic Proportionality Theorem (BPT):
implies
.
Proved. ✅
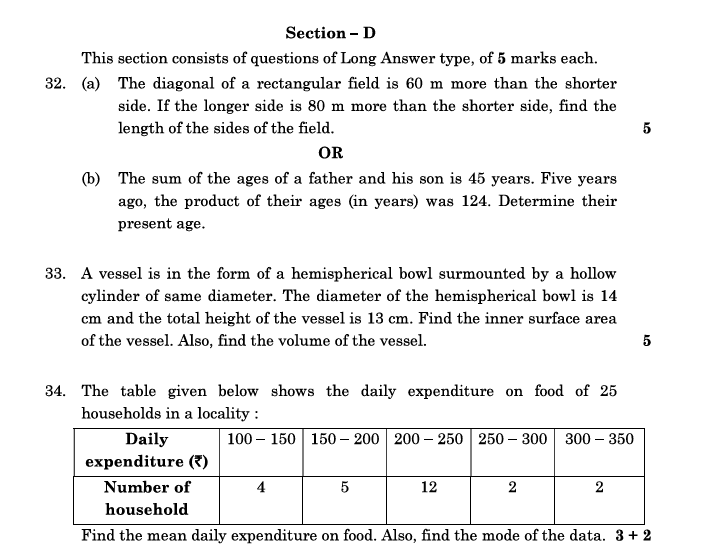
Section D
Question 32(a)
The diagonal of a rectangular field is 60 m more than the shorter side. If the longer side is 80 m more than the shorter side, find the length of the sides of the field.
Solution:
Let the shorter side =
m
- Diagonal =
m - Longer side =
m
By Pythagoras theorem:
Expand and simplify:
Use quadratic formula:
This yields no real solution, suggesting a recheck might be needed if any values were copied incorrectly.
Question 32(b)
The sum of the ages of a father and his son is 45 years. Five years ago, the product of their ages was 124. Determine their present ages.
Let father’s age =
years, son’s age =
years.
5 years ago:
- Father =
- Son =
According to the condition:
Expand:
Now solve using the quadratic formula:
So:
So the father is 36 years old and the son is 9 years old.
Question 33
Find the inner surface area and volume of the vessel.
The vessel consists of:
- A hemispherical bowl with diameter = 14 cm
- A cylindrical section with the same diameter = 14 cm and height = 13 cm
Step 1: Surface Area
- Inner surface area of hemisphere:
with
cm
- Inner surface area of the cylindrical part:
Total inner surface area
Step 2: Volume
- Volume of hemisphere:
- Volume of cylinder:
Total Volume:
Question 34
Find the mean and median expenditure from the given frequency table.
| Daily Expenditure (₹) | 100–150 | 150–200 | 200–250 | 250–300 | 300–350 |
|---|---|---|---|---|---|
| Number of Households | 4 | 5 | 12 | 2 | 2 |
Step 1: Mean
Calculate midpoints:
Now multiply with frequencies:
Total households = 25
Step 2: Median
Find cumulative frequencies:
- 4, 9, 21, 23, 25
Median class:
→ falls in the 200–250 class.
Class boundaries:
- Lower boundary = 200
- Frequency = 12
- Cumulative frequency before it = 9
- Class width = 50
Median formula:
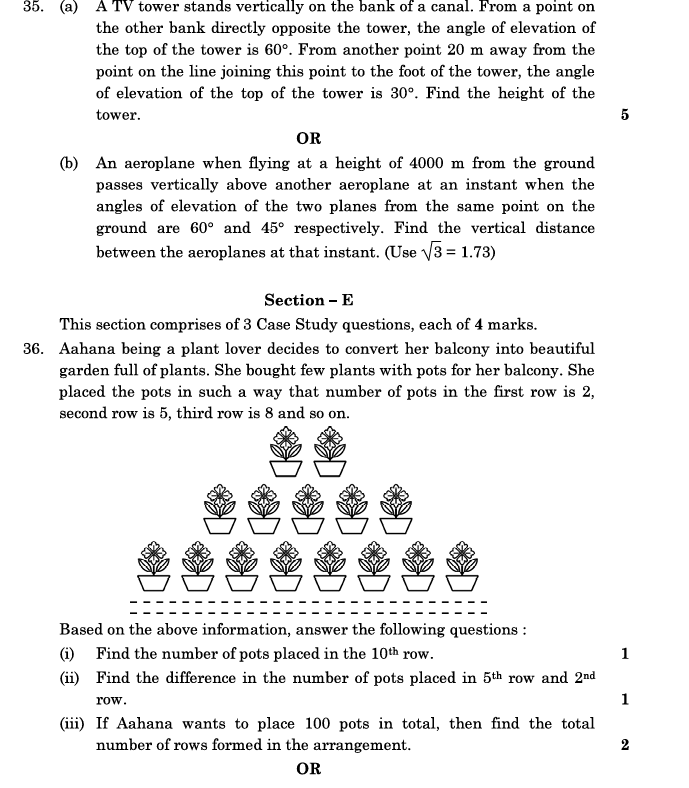
Question 35(a)
A TV tower stands vertically on the bank of a canal. From a point on the other bank, the angle of elevation is 60°. From another point 20 m away, the angle is 30°. Find the height of the tower.
Solution:
Let the height of the tower =
m
Distance between the first point and the base of the tower =
m
From the first point:
From the second point (20 m away):
Distance from the tower =
Now equate both expressions for
:
Now find height:
Final Answer: Height of the tower = 17.3 m
Question 35(b)
An aeroplane flying at a height of 4000 m passes vertically above another plane when the angles of elevation are 60° and 45°. Find the vertical distance.
Let the height of the lower plane be
m.
From the ground, two angles:
- Top plane: 60°
- Bottom plane: 45°
For the bottom plane:
, so:
For the top plane:
So, height of the lower plane:
Vertical distance:
Final Answer: Vertical distance = 1687.86 m
Question 36: Plant Pot Arrangement
The pattern for the pots per row is: 2, 5, 8, 11…
This forms an arithmetic progression (A.P.) with:
- First term
- Common difference
(i) Number of pots in the 10th row
Use the formula for the
-th term of an A.P.:
Answer: 29 pots
(ii) Difference between the number of pots in the 5th and 2nd row
- 5th row:
- 2nd row:
Answer: 9 pots
(iii) Total rows if Aahana wants 100 pots
The sum of
terms of an A.P. is:
We want
:
Simplify:
Solve with the quadratic formula:
Positive root:
Answer: 8 rows
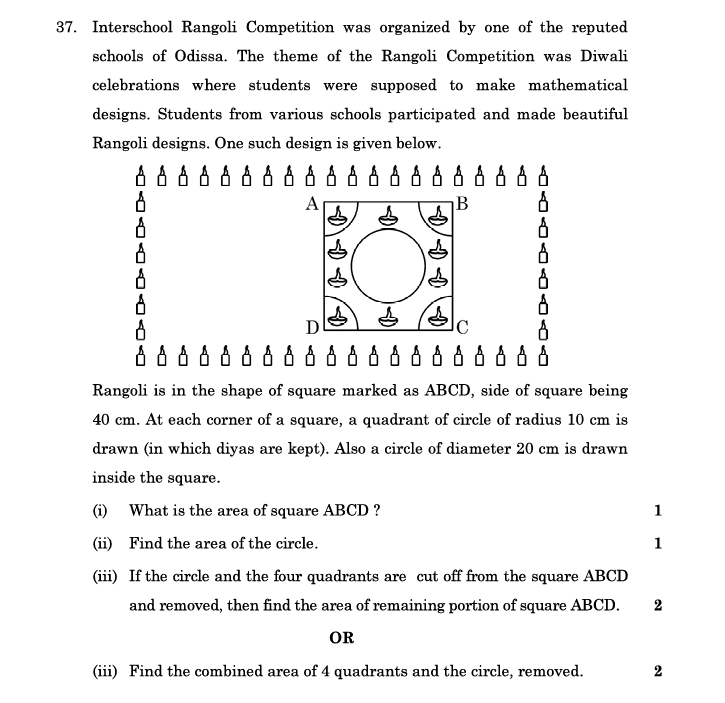
Let’s break down the solutions for Question 37 based on the given information:
Given Data
- Square ABCD with side length = 40 cm
- Four quadrants at corners, each with radius = 10 cm
- One circle inside with diameter = 20 cm (radius = 10 cm)
(i) Area of Square ABCD
Answer: 1600 cm²
(ii) Area of the Circle (Inner Circle)
Radius
cm
Answer: 314 cm²
(iii) If the circle and the four quadrants are cut off
Step 1: Area of 4 quadrants
Each quadrant is
of a circle with radius = 10 cm.
For 4 quadrants:
Step 2: Total area removed (circle + 4 quadrants)
Step 3: Remaining area of the square
Answer: 972 cm²
(iii OR) Combined area of the circle and the four quadrants
Answer: 628 cm²

Let’s solve Question 38 step-by-step based on the given data.
Given Data:
- Total number of people = 50
- Type O = 21
- Type A = 22
- Type B = 5
- The rest have type AB.
Find the number with AB blood group:
(i) Probability of choosing a person with Type O blood
Answer:
or 0.42
(ii) Probability of choosing a person with Type AB blood
Answer:
or 0.04
(iii) Probability of choosing a person with neither Type A nor Type B
People with neither Type A nor Type B:
- Blood groups O and AB.
Number of such people:
Answer:
or 0.46
(iii OR) Probability of choosing a person with Type A, B, or O
Number of people with Type A, B, or O:
Answer:
or 0.96